Springing Simulations Forward with Quantum Computing
Simulating oscillator dynamics is now faster with quantum computing
April 11, 2024
 enlarge
enlarge
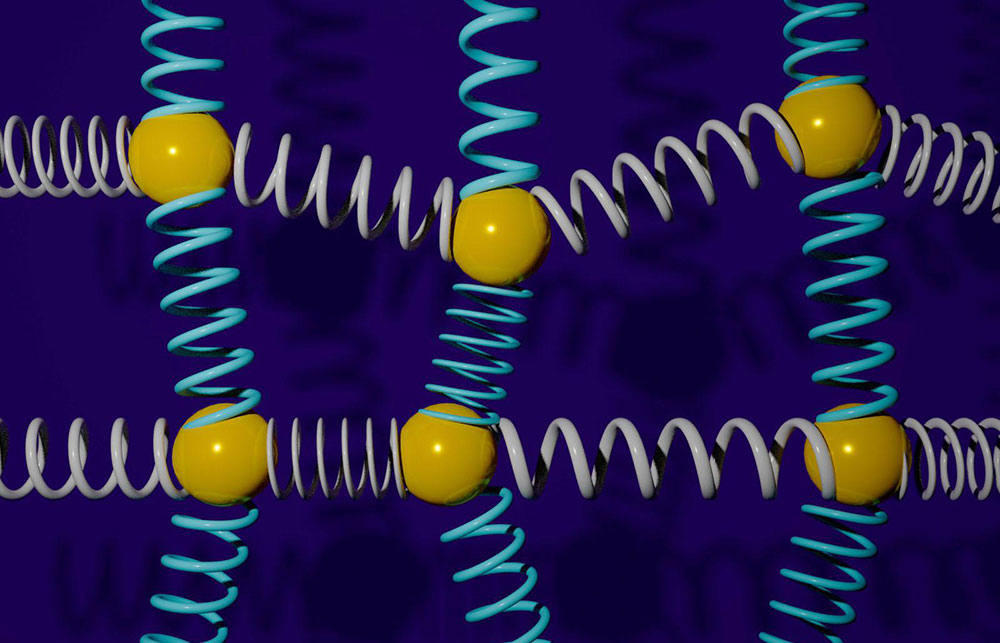
The dynamics of coupled oscillators, such as those shown here, can be simulated faster with a new quantum algorithm. (Image by Nathan Johnson | Pacific Northwest National Laboratory)
Editors' note: The following feature story was originally issued by the U.S. Department of Energy’s (DOE) Pacific Northwest National Laboratory (PNNL). PNNL is a partner institution of the Co-design Center for Quantum Advantage (C2QA), a National Quantum Information Science Research Center funded by the DOE Office of Science. DOE’s Brookhaven National Laboratory is the lead institution for C2QA. Nathan Wiebe leads C2QA’s Software & Applications Thrust. For more information on Brookhaven’s involvement, contact Danielle Roedel (droedel@bnl.gov).
Though “coupled oscillations” may not sound familiar, they are everywhere in nature. The term 'coupled harmonic oscillators' describes interacting systems of masses and springs, but their utility in science and engineering does not end there. They describe mechanical systems like bridges, the bonds between atoms, and even gravitational tidal effects between the Earth and the Moon. Understanding such problems allows us to probe a correspondingly huge range of systems from chemistry to engineering to materials science and beyond.
Classically represented by a ball and spring model, coupled oscillatory systems become increasingly complex as more oscillators are added. With a new quantum algorithm created in part by Pacific Northwest National Laboratory (PNNL) joint appointee and University of Toronto Professor Nathan Wiebe, simulating such complex coupled oscillator systems is now faster and more efficient. These results were published in Physical Review X.
Partnering with researchers from Google Quantum AI and Macquarie University in Sydney, Australia, Wiebe developed an algorithm for simulating systems of coupled masses and springs on quantum computers. The researchers then provided evidence of the new algorithm’s exponential advantage over classical algorithms.
This speedup was made possible by mapping the dynamics of the coupled oscillators to a Schrödinger equation—the quantum counterpart to a classical Newtonian equation. From there, the system could be simulated using Hamiltonian methods. In essence, this approach allows scientists to express the dynamics of coupled oscillators using far fewer quantum bits than traditional methods. Researchers can then simulate the system using exponentially fewer operations.
Perhaps the most intriguing aspect of their work arises from the question of whether this algorithm indeed offers an exponential speedup over all possible ordinary algorithms. First, the authors showed that this algorithm works both ways: that coupled harmonic oscillators can be used to simulate an arbitrary quantum computer. This means that, at a high level, very large systems of interacting masses and springs can contain within them computational power equivalent to a quantum computer.
Second, the authors considered the theoretical constraints around calculating these dynamics. If there existed a way to simulate these dynamics in polynomial time on existing computers, then researchers could construct a faster method for simulating quantum computers. However, this would prove that quantum computers are essentially no more powerful than classical computers. Evidence accumulated over the years shows that it is exceptionally unlikely that classical computers are qualitatively as powerful as quantum computers. Thus, this work provides a convincing argument that this algorithm affords an exponential speedup as well as a clear demonstration of a novel and subtle link between quantum dynamics and the humble harmonic oscillator.
“Very few new classes of provable exponential speedups of classical calculations have been developed,” said Wiebe. “Our work provides a significant computational advantage to a wide range of problems in engineering, neuroscience and chemistry.”
This work was supported by the Department of Energy, Office of Science, National Quantum Information Science Research Centers, Co-design Center for Quantum Advantage (C2QA) (PNNL FWP 76274). This work was also supported by grants from Google Quantum AI and the Australian Research Council.
2024-21834 | INT/EXT | Newsroom